Larger Photograph (11.4K)

Larger Photograph (61.5K)

Larger Photograph (102.4K)

Larger Photograph (59.4K)


| Designer | : | Unknown | ||
| Craftsman | : | Stewart Coffin (1st) Stewart Coffin (2nd) Tom Lensch (3rd & 4th) Stewart Coffin (5th) Jerry McFarland (6th) Interlocking Puzzles (7th) | ||
| Category | : | Interlocking | ||
| Material | : | Cherry (1st & 2nd) Mahogany (3rd & 4th) Cherry (5th) Tulipwood (6th) | ||
| Pieces | : | 6 | ||
|
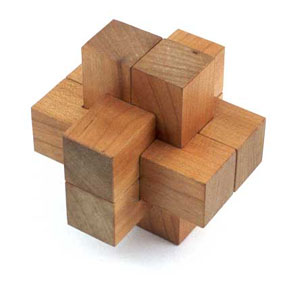
Without a doubt, the six-piece burr is the most familiar burr. There is not a single six-piece burr puzzle, but billions of them. How the pieces are notched and their length determine how each is different from the other. Several versions have been patented and manufactured. The earliest US patent is No. 1,225,760 of Brown, dated 1917, with several others following shortly thereafter.
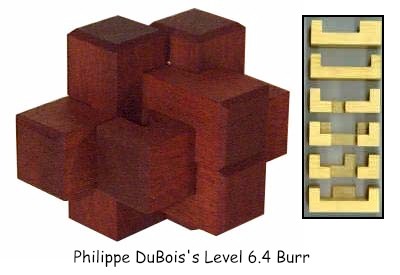
Six-piece burrs can be found in many toy and game stores today, but they are almost always copies of versions with sliding keys and internal symmetries that have been available for many years. The six-piece burr's simple appearance and the fact that the simplest one looks identical the the most complicated causes it to be ignored by those unfamiliar. Bill Cutler published at article titled The Six-Piece Burr in the Journal of Recreational Mathematics, Vol 10(4), 1977-78, pp. 241-250 which described the solid six-piece burr completely. He showed there are twenty-five possible notchable pieces to make solid six-piece burrs and that they can be put together in 314 ways. He also showed there are 369 general pieces usable to make solid burrs and that they can be put together in 119,979 ways. Shown in the 1st photograph is Burr Number 305. This is the burr that ended up with number 305 in Bill Cutler's analysis. It has an unusual level 3.3 solution. Stewart made about 60. Number 35 in his numbering system. The 2nd and 3rd photographs are Philippe Dubois's level 6.4 (six moves to remove the first piece and four to remove the second) burr. The 4th and 5th photographs are Peter Marineau's unique Level 9 burr. It was named the Piston Puzzle because of the unusual movement of the pieces during assembly and disassembly. Peter came up with this design by hand in 1986. It surpassed the previous highest level by Philippe Dubois. In 1987, Bruce Love came up with Love's Dozen, a nonunique level 12 burr. The Piston Puzzle held top honors for unique solutions until Bill Cutler's computer analysis of all six-piece burrs produced The Unique 10 and others. The 6th photograph is Wayne Daniel's Forty-Two-Piece Burr Set that contains the twenty-five notchable pieces (up to three of each) that are required to build the 314 solid, notchable burrs. The term "notchable" is used to describe pieces which can be made with a table saw instead of those which have blind corners and inside edges which must be chiseled out. And "solid" means the puzzle has no internal voids when assembled. These solid, notchable six-piece burrs are considered to be the "purest" form of the six-piece burr. Because there are no internal voids, at least one piece must come out with a single move. Of the 314 solid, notchable burrs, 158 begin with the removal of the piece with no notches. For the other 156, at least two pieces must be removed on the first move. A complete description of the six-piece burr would be a web site all of it's own. And, in fact, such a site exists. It was developed by Jürg von Känel at IBM Research. His Burr Puzzles site contains a Java application that allows you to see, through Java controlled animation, how many of the well-known six-piece burrs, all of the 314 solid notchable burrs and many others work. And you can create your own and have the application compute all of the assemblies (if there are any) and animate the solutions. You might not make it back!
| ||||
