
Larger Photograph (75.3K)

| Designer | : | Stewart Coffin | ||
| Craftsman | : | Stewart Coffin (1st & 2nd) Walter Hoppe (3rd) | ||
| Category | : | Put-Together | ||
| Material | : | Various (1st & 2nd) Cherry & Walnut (3nd) | ||
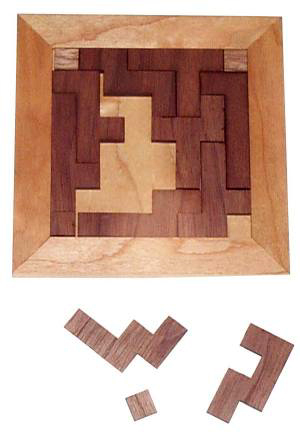
| Pieces | : | 14, plus 1 2X2 square and the tray | ||
|
If you take the thirty-five possible hexominoes and eliminate those wth symmetry and those which are non-elongate (containing a 2X2 square), seventeen remain.
Stewart's Cornucopia puzzles are made by selecting ten of the seventeen pieces. The 2nd Cornucopia puzzle (Number 101715) has a unique solution when a 1X1 square occupies the four corners and a unique solution when the 2X2 square occupies the center location. Walter built the frame double-sided so the owner can glue the 1X1 and 2X2 squares into place if so desired. Using Bill Cutler's box-filling program, Walter found eight solutions where the extra 2X2 square filled other than the center location and twenty-nine solutions where the 1X1 squares are in locations other than the corners. The ten hexomino pieces also make a 6X10 rectangle. This is a very difficult puzzle.
| ||||
